3.1. Solitary Wave Propagation over a Continental Shelf with Periodic Lateral Variation
Numerical results are presented for a solitary wave propagating over a continental shelf with a ridge-valley configuration as seen in the planview image presented in
Figure 1. The bathymetry is given by:
where the height of the step is
, the steepness in the
direction is
, and the amplitude of the lateral perturbation in the bathymetry is
. In order to ensure periodicity in the
direction, we set
: namely the breadth between valleys is
. The bathymetry is translated to the right of the origin by an amount of
so that the initial wave form is not disturbed by the presence of the continental shelf. Note that our coordinate system is oriented such that
x is in the dominant direction of wave propagation where the origin
gives the initial location of the crest of the solitary wave, and
y is the spatial coordinate in the lateral direction. The bathymetry is symmetric about the origin
on account of the spatial periodicity in the
direction. Because of this periodicity, from hereinafter, the numerical results are presented only in the span
, and we call the bathymetry presented in (
12) the 2D case, while the bathymetry with no lateral variation will be referred to as the 1D case.
The spatial resolutions in the
and
directions are chosen to be
and
, respectively. A time step of
is used in this simulation and we truncate the asymptotic series (
3) to
terms. We include
and
modes in the basis functions (
4) and (
5).
The initial conditions are prescribed by the third-order solitary-wave solution by Grimshaw [
22] where the initial water-surface displacement
and velocity potential
are given by:
where
S and
T are:
in which
. In this experiment, the initial wave amplitude is
. Recall that
is the wave amplitude normalized by the offshore still water depth and is therefore a measure of the initial nonlinearity of the wave.
In
Figure 2, we present snapshots of the water-surface displacement for the solitary wave evolving over the bathymetry specified by (
12). In
Figure 2a, we present the initial offshore solitary-wave conditions. Then at time
(
Figure 2b), the effects of refraction begin to emerge from the presence of the bathymetry, creating a phase difference between the waveform at the ridge (
) and at the valley (
). Note that soon after refraction occurs, a stem-wave formation appears in the vicinity of
, which is similar in form to the wave pattern created by obliquely interacting solitary waves in a uniform depth: see for example, Yeh & Li [
23] and Kodama & Yeh [
24]. Due to the formation of “radiating” waves from the stem, energy is allowed to leak out laterally which is then displaced behind the lead waveform.
At time
(
Figure 2f), we observe a negative difference in spatial phase lag (
) even though the local water depth is shallower at
than at
. Note that the spatial phase difference is defined as
, where
gives the
coordinate of the lead crest of the solitary wave at
, and
gives the
coordinate of the lead crest at
. This negative phase lag is also observed in
Figure 2g,h. While the negative phase lag is present, energy is allowed to travel outwards in the lateral directions, transverse to the direction of wave propagation, which then accumulates at
, at the valleys of the bathymetry. This then commences in an increase in amplitude and thus phase speed at the valleys, which leads to a positive spatial phase difference (
) as can be observed at
(
Figure 2i). In
Figure 2j,k we continue to see a reciprocating behavior in the spatial phase lag where the middle of the waveform bends forwards and backwards in time. However, as the wave climbs up the shelf, the waveform is tending towards an asymptotic state where the solitary wave is recovered and the crest of the wave becomes straight and parallel to the shoreline. Note that the waveform at time
(
Figure 2k) is of greater amplitude, but of narrower width, than the initial solitary wave. The total mechanical energy of the initial solitary wave is compared with the lead solitary wave at the end of the numerical simulation, and the ratio of the final-stage energy to the initial energy was found to be 0.74. This is the amount of energy reduction in the leading wave due to dispersion/radiation effects which took place over the wavy bathymetry.
In
Figure 3a, we present a portion of the domain along the ridge (
) at time
. The waveform over the plateau region possesses a lead solitary wave and trailing waves of smaller amplitude. In order to assess if these are truly solitary waves, we compare the computed numerical water-surface displacement with the higher-order solution (
13) by Grimshaw [
22]. An overlay presented in
Figure 3 shows that the lead and intermediate waves are solitary waves. However, the third wave, presented in
Figure 3d, does not appear to be a clean solitary wave.
In
Figure 4, we present the amplitude variation along the ridge
and the valley
. In addition, the result from the 2D case is compared with the 1D wave-propagation case which will be discussed later in
Section 3.1.1. The amplitude at
starts to increase at the toe of the slope (
) and reaches a maximum of
at
. The amplitude at
then attains a minimum value of
at
. We observe a “saw-tooth” pattern where the amplitude at
oscillates out of phase with the amplitude at
. The amplitude appears to be approaching an asymptotic mean state of
for the 2D case, whereas the amplitude for the 1D case is approaching a larger value of
. This smaller amplitude for the 2D case is due to energy dispersion effects resulting from the creation of radiating waves in the vicinity of
.
The spatial phase difference
is presented in
Figure 5. Again, we see an oscillatory behavior in the phase lag which also appears to be tending towards an asymptotic state:
as
. The maximum positive value of phase lag is
at the location
. The most negative value of phase lag is
at the location
. Note that, the greatest absolute difference in phase lag occurs when the waveform in the shallower region of the domain is moving faster than the waveform in the deeper portion of the domain.
3.1.1. Solitary Wave Propagation over a Continental Shelf with no Lateral Variation
For comparison, we run a numerical simulation for the 1D wave-propagation case. The initial wave conditions are given by equation (
13) where the amplitude is
and the initial location of the crest is
. The bathymetry with no lateral variation is now given by:
The parameters for the bathymetry (
15) are
,
,
, and
. Note that this is the same bathymetry as for the 2D wave-propgation case (
12) if we had chosen
. A temporal and spatial resolution of
and
are used, respectively. We include
terms in the perturbation expansion and truncate the basis functions to
modes.
In
Figure 6, we provide snapshots of the free-surface displacement along with the corresponding bathymetry at various times.
Figure 6a shows the initial condition of the waveform at the offshore location. At time
(
Figure 6b), the solitary wave has encountered the increasing bed elevation and the water-surface displacement loses its symmetry. The amplitude increases and the solitary wave becomes narrower. In
Figure 6c, we observe the presence of a trailing wave resulting from fissioning that occured after the solitary wave climbed onto the plateau region of the bathymetry. The solitary waveform is then recovered as demonstrated in
Figure 6d with a larger amplitude and narrower width than the initial solitary wave.
The waveform is presented in
Figure 7 at time
. We see a similar pattern in the water-surface displacement along
for the 2D case seen in
Figure 3. However, for the 2D case, there are extra trailing waves. As we stated earlier, the amplitude of the lead solitary wave is larger for the 1D case than for the 2D case. Furthermore, in
Figure 7, we overlay the numerical result with the higher-order solitary-wave solution (
13) by Grimshaw [
22] in order to check if the trailing and leading waves are solitary waves. It appears that the first two leading waves, as seen in
Figure 7b,c are solitary waves, but the trailing wave, as seen in
Figure 7d is similar to a solitary wave, but not quite the exact same form. Therefore, just as in the 2D case, it appears that there are two solitary waves that emerge from the fissioning process. The numerical result for the number of solitons which emerge is compared with the analytical prediction presented in (Johnson [
25] Section 3.4.4):
which gives a value of
for
. Our numerical results support the analytical prediction (
16). Note that, in the present case we examined, the analytical prediction coincides with the numerical result even for the 2D bathymetry.
3.2. Undular Bore Propagation over a Continental Shelf with Periodic Lateral Variation
We now examine an undular bore propagating over a bathymetry representing a continental shelf with a laterally-periodic ridge-valley configuration, similar to what is seen in
Figure 1. The initial wave condition is prescribed by a smooth step-shaped displacement with zero velocity. The initial water-surface displacement and velocity potential are given, respectively, by:
where
and
. The bathymetry is prescribed by (
12) where the parameters are given by
,
,
, and
. In this simulation we choose
in order to give the initial wave condition ample time to evolve into an undular bore before encountering the continental shelf. We set a time step at
and the spatial resolutions in the
and
directions are
and
, respectively.
terms are included in the perturpation series, and the modes in the
and
directions are truncated to
and
, respectively.
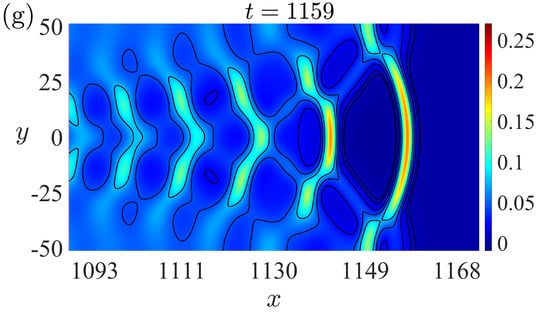
Figure 8 shows snapshots of the plan view of the water-surface displacement along with the corresponding bathymetry. In
Figure 8a, we see the initial offshore water-surface displacement at
. The smooth step then evolves into an undular bore in the offshore region as shown in
Figure 8b. At time
(
Figure 8c), the undular bore encounters the toe of the continental slope and refracts about the crest of the ridge. In
Figure 8d,e, the lead crest of the undular bore begins to straighten out while “radiating” waves emerge from the ends of the stem; this is similar to the pattern observed in the solitary wave case, as discussed in
Section 3.1. At time
(
Figure 8f), it appears that the center of the waveform begins to bow forward which continues in
Figure 8g,h. At time
, (
Figure 8i), the amplitude at the valley (
) becomes greater than at the ridge. In
Figure 8j, solitons disintegrate from the front of the undular bore, resulting in a total of five solitary waves that have emerged at time
as shown in
Figure 8k.
Figure 9 shows the spatial evolution of the lead-wave amplitude of the 2D undular bore against location. The amplitude at the ridge (
) is represented by a solid line and the amplitude at the valley (
) is represented by a dashed line. The dotted line represents the amplitude of the lead crest of the undular bore for the 1D case which is discussed later in
Section 3.2.1. The amplitude at
is
, which represents the height immediately after the intial water-surface displacement is set in motion. This initial height is in agreement with the prediction given by the method of characteristics for the nonlinear-nondispersive shallow-water-wave equation for a dam-break initial condition:
Soon after, the amplitude of the lead wave of the undular bore slowly increases until reaching
. Once the undular bore encounters the sloping bed, we see that the amplitude at the ridge (
) quickly increases, reaching a maximum value of
at
, and the amplitude in the valley
decreases, reaching a minimum value of
at
. The amplitude then behaves in a reciprocating fashion, creating a saw-tooth pattern. The amplitude at
is roughly out of phase with the amplitude at
. It appears though that the amplitude may approach an asymptotic state given a sufficient amount of time. For the 1D case that is represented by the dotted line in
Figure 9, the amplitude increases monotonically until reaching the asymptotic value of
after reaching the plateau. It appears that the amplitudes for the 1D and 2D cases become similar in the limit as
. Recall that the amplitudes for the 1D and 2D cases of the solitary waves are different (
for the 1D case and
for the 2D case) as
.
The spatial phase lag
is presented in
Figure 10 for the 2D undular bore case. The phase lag remains at
until encountering the toe of the bed slope at
. At this point, a positive phase lag is created from the undular bore refracting about the crest of the continental shelf. A maximum value of
is attained at
. However, the spatial phase lag then sharply decreases to a minimum value of
at
. It appears that the phase lag is approaching an asymptotic value such that
as
. Note that, unlike the solitary wave case (
Figure 5), the magnitudes of the maximum and minimum phase lags are similar for the undular bore.
3.2.1. Undular Bore Propagation over a Continental Shelf with no Lateral Variation
For comparison, we perform numerical experiments where an undular bore propagates over a smooth step into shallower waters without lateral variation (see the sketch in
Figure 11). The same initial conditions along
as (
17) is applied. In this numerical experiment, we set
,
,
, and
.
Snapshots of the entire domain are presented in
Figure 12. The initial conditions are shown in
Figure 12a. The smooth step then evolves into an undular bore and a dispersive rarefaction wave with an expanding mean water depth, which separates the two ends of the waveform. The mean water depth is equivalent to the height predicted by Equation (
18). Once encountering the toe of the continental slope, the undular bore shoals, and a careful observation in
Figure 12c reveals the occurrence of energy reflection at time
. Note that, at the beginning of the simulation, there are only two mean water depths. However, after the undular bore climbs up and passes over the sloping region, five distinct mean water depths can be identified at
as shown in
Figure 13. We may roughly partition these depths into the following regions: (1):
for
; (2):
for
; (3):
for
; (4):
for
; and (5):
for
. Regions (1), (2), and (5) are relatively straightforward to interpret. However, regions (3) and (4) require some explanations. Within region (4), the velocity field reaches an essentially steady flow condition. This is supported by
Figure 14 which displays the depth-averaged horizontal velocity
plotted against time at locations
and
. After about
, the velocity appears to maintain the steady state. Note that the depth-averaged horizontal velocity is defined as
in which
u is the horizontal fluid velocity and
is the local total water depth. The fluid velocities are normalized by
. The vertical component of the velocity is essentially nil. For an inviscid and homogeneous fluid, the total energy head (total mechanical energy plus pressure work per unit fluid weight) must be conserved. Therefore:
where
and
are the specific energy before and after the bed slope, respectively, which are defined as:
where the subscripts 3 and 4 refer to the values in the regions 3 and 4, respectively, (see
Figure 13), and
H is the total water depth. Following this notation, the height of the step can be given by the difference in the bed elevations:
. From Equations (
20) and (
21), the theoretical total water depth at region 4 can be computed and found to be 0.556. This is calculated from the numerical data attained at
with
,
,
, and
. The actual value attained from the numerical experiment was
, resulting in no error up to the first three decimal places.
The analysis between regions (3) and (4) indicates that the flow is subcritical relative to the laboratory coordinate, and a standard analysis of the specific energy in the area of open-channel flows (see e.g., Henderson [
26]) can be applicable here for the quasi-steady flow condition.